Order of Operations

In every walk of life we are met with a set of orders or patterns without which everything falls apart. Just like when we get up in the morning we brush our teeth, finish our morning ablutions, eat breakfast, pack our stuff and leave for school. We cannot afford to mess the order because then it gets absurd.

In the same way in Mathematics a principle for order of operations is followed.“Operations“ means to add, subtract, multiply, divide, squaring, etc. If it isn’t a number it is probably an operation.

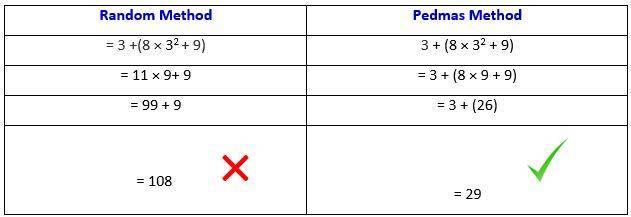
But if we came across: 3 + (8 × 3² + 9) how should we calculate?

Will left to right solution get us the answer?
Will right to left lead to the right answer?
Will solving the exponents first and then the rest get us to the end?

All across the globe mathematicians were completely confused with the respected results.

Hence, they came up with a conclusion to follow an order to operate a set of parameters.

The order of operations is the order which is followed to add, subtract, multiply or divide to solve a problem in mathematics.

Order of operations is the method which operations should be performed in a particular order. It gives us a procedure as to which operations to do first.

To remember the conventional order of operations, you can think of –

PEMDAS (“Please excuse my dear Aunt Sally” is an easy way to memorize this)

Parentheses
Exponents
Multiplication and Division
Addition and Subtraction

This means that first we should do what data is within parentheses, then exponents, followed by multiplication and division always from left to right), and then addition and subtraction (from left to right). If parentheses are within other parentheses, solve it from the inside out.

Coming back to the above example and why is it necessary to follow the order PEDMAS.

Check Point

Now try to answer the following:

29 – 23 × 0 ÷ 1
65 ÷ 5 × 7 × 3
19 – 12 + 4 × 3
4 × 4 ÷ 1 + 9
20 – 10 × 3 ÷ 1

Answer key

0
273
19
25
30

Personalized Online Tutoring

eTutorWorld offers affordable one-on-one live tutoring over the web for Grades 2-12, Test Prep help for Standardized tests like SCAT, CogAT, SSAT, SAT, ACT, ISEE and AP. You may schedule online tutoring lessons at your personal scheduled times, all with a Money-Back Guarantee. The first one-on-one online tutoring lesson is always FREE, no purchase obligation, no credit card required.

For answers/solutions to any question or to learn concepts, take a FREE Demo Session.

Schedule a Free Session

No credit card required, no obligation to purchase.
Just schedule a FREE Sessions to meet a tutor and get help on any topic you want!

Pricing for Online Tutoring

Tutoring Package	Validity	Grade (1-12), College
5 sessions	1 Month	$129
1 session	1 Month	$26
10 sessions	3 months	$249
15 sessions	3 months	$369
20 sessions	4 months	$469
50 sessions	6 months	$1099
100 sessions	12 months	$2099