Dot Plots
Grade 6 Math Worksheets
Data is the foundation upon which insights and conclusions are built in mathematics. Visualizing data is an art that transforms numbers into stories, and one of the most elegant and intuitive ways to do so is through dot plots. Dot plots are a minimalist yet powerful tool that allows us to represent data point by point, revealing patterns, trends, and distributions with remarkable clarity.
List of Contents:
- The Essence of Dot Plots
- Constructing a Dot Plot
- Understanding Dot Plots
- Practical Application
- Reading a Dot Plot
- Types of dot plots
- Step-by-Step Analysis
- FAQs
Personalized Online Tutoring
Dot Plots - Grade 6 Math Worksheet PDF
This is a free printable / downloadable PDF worksheet with practice problems and answers. You can also work on it online.
Sign up with your email ID to access this free worksheet.
"We really love eTutorWorld!"
"We really love etutorworld!. Anand S and Pooja are excellent math teachers and are quick to respond with requests to tutor on any math topic!" - Kieran Y (via TrustSpot.io)
"My daughter gets distracted easily"
"My daughter gets distracted very easily and Ms. Medini and other teachers were patient with her and redirected her back to the courses.
With the help of Etutorworld, my daughter has been now selected in the Gifted and Talented Program for the school district"
- Nivea Sharma (via TrustSpot.io)
The Essence of Dot Plots:
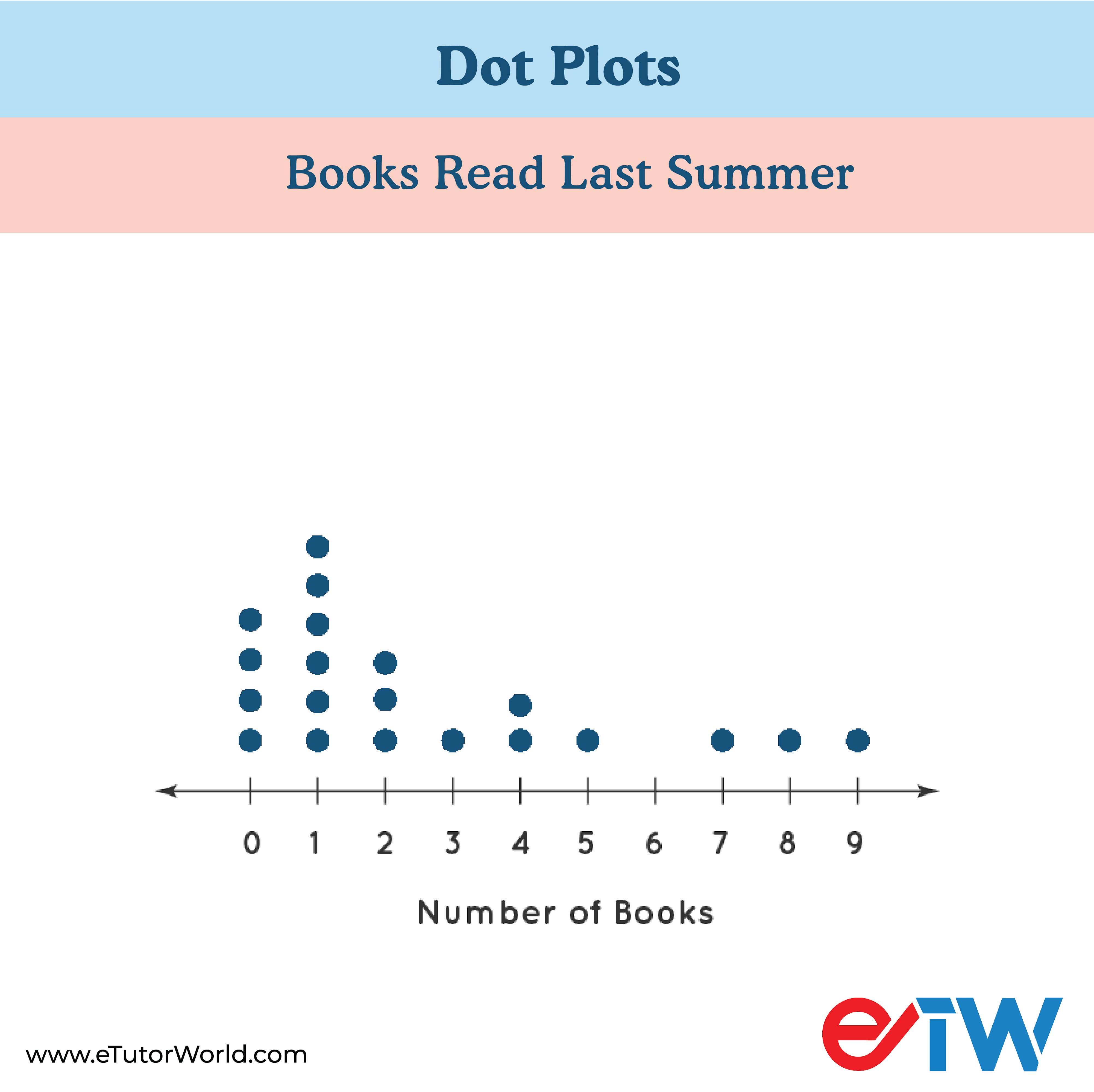
Imagine taking a magnifying glass to your data, focusing on each individual data point. It is the essence of a dot plot. At its core, a dot plot showcases data points as individual dots along a single axis. This minimalist approach distills complex datasets into a visual representation that highlights the frequency and distribution of values. Dot plots are the storytellers of the data world, portraying each data point’s unique journey in an elegant and enlightening way.
Constructing a Dot Plot:
Creating a dot plot is straightforward:
Choose an Axis: Select a numerical axis that suits your data. It could represent time, age, test scores, or any other relevant variable.
Place Dots: Place a dot above the corresponding value on the axis for each data point. If multiple data points have the same value, stack the dots vertically.
Labeling and Scaling: Label the axis appropriately and ensure the scale accurately represents the data values.
Adding Context: Provide a title and labels for the axis to offer context and aid interpretation.
Understanding Dot Plots:
Dot plots offer a multitude of benefits for understanding data:
Individual Insight: Each dot represents a unique data point, allowing you to see the distribution point by point.
Frequency: The density of dots at specific values indicates the frequency of occurrence.
Outliers: Outliers, or data points significantly different from others, stand out clearly.
Comparisons: Dot plots are excellent for comparing multiple datasets side by side.
Practical Application:
Dot plots find their home in various real-world scenarios:
Classroom Scores: Analyze test scores to identify the most common scores and outliers.
Population Age Distribution: Visualize the age distribution of a population to understand the age group’s prevalence.
Product Ratings: Represent customer ratings to see which are most frequent and rare.
Reading a Dot Plot
Reading a dot plot involves understanding its components and extracting insights about its data distribution. Here is a step-by-step guide on how to read a dot plot:
1. Identify the Axis: Locate the axis along which the data values are plotted. This axis represents the data values you analyze, such as test scores, ages, or any other numerical variable.
2. Observe the Dots: Each dot on the dot plot represents an individual data point. Pay attention to the position of the dots along the axis.
3. Frequency of Dots: The density of dots at a specific point on the axis indicates the frequency of occurrence of that value in the dataset. More densely-packed dots represent higher frequency.
4. Horizontal or Vertical Stacking: If multiple data points have the same value, they might be stacked horizontally or vertically. This stacking technique helps visualize the frequency of repeated values.
5. Central Tendency: Observe where the majority of dots are clustered. It can give you a sense of the central tendency of the data.
6. Spread and Distribution: Pay attention to how widely spread the dots are along the axis. A wider spread indicates a greater variability in the data values.
7. Outliers: Look for any dots that are isolated from the main cluster. These isolated dots could be outliers, which are data points significantly different from others.
8. Comparative Analysis: If you compare multiple dot plots side by side, compare the frequencies, central tendencies, and distributions to identify differences and similarities between datasets.
9. Contextual Interpretation: Consider the context of your analyzing data. For example, suppose you are looking at test scores. In that case, you might identify common scores, understand the spread of scores, and identify exceptional performance or underperformance.
10. Drawing Conclusions: Based on your observations, conclude the data distribution. Is the data clustered around certain values? Is it spread out or tightly packed? Are there outliers that need further investigation?
11. Communicate Findings: If you are presenting your analysis, communicate your findings effectively. Use the insights from the dot plot to support your conclusions and insights.
12. Remember the Scale: Pay attention to the scale of the axis. The distance between values can impact the perception of frequency and distribution.
13. Contextualize Outliers: If you identify outliers, consider their potential significance. Are they data errors, anomalies, or indicative of a unique phenomenon?
“There have been times when we booked them last minute, but the teachers have been extremely well-prepared and the help desk at etutorworld is very prompt.
Our kid is doing much better with a higher score.”
6th Grade Tutoring
eTutorWorld offers Personalized Online Tutoring for Math, Science, English, and Standardised Tests.
Our Tutoring Packs start at just under $22.49 per hour, and come with a moneyback guarantee.
Schedule a FREE Trial Session, and experience quality tutoring for yourself. (No credit card required.)
Types of dot plots
1. Basic Dot Plot: The foundational type of dot plot features individual data points represented by dots along a single axis. The density of dots at each value highlights frequency, making it easy to identify common values and outliers. Basic dot plots are ideal for datasets where individual data points hold significance.
2. Strip Plot: A variation of the basic dot plot, the strip plot places dots vertically at exact values. This form is particularly useful when data points have unique identifiers, allowing easy tracking and interpretation. Strip plots are commonly used in research and clinical studies to display measurements of individual subjects.
3. Stacked Dot Plot: Stacked dot plots are employed when multiple datasets or categories must be compared. Here, dots representing different categories are stacked vertically or horizontally. This type is excellent for visualizing distributions across subgroups, such as test scores for different subjects.
4. Dot Density Plot: In situations where a large dataset needs to be visualized, dot density plots come into play. Rather than representing individual data points, these plots show the density of points within specific ranges. Dot density plots are perfect for highlighting overall trends while maintaining clarity with a sizable dataset.
5. Quantile Dot Plot: Quantile dot plots provide insights into the distribution of data across percentiles. Each dot represents a data point, and their arrangement indicates the distribution’s shape and spread. These plots are insightful for understanding data percentiles and identifying quartiles.
6. Categorical Dot Plot: For categorical data, where data points belong to distinct categories, categorical dot plots showcase the frequency of each category. This type of plot is effective in revealing the popularity or prevalence of different options in survey responses or preferences.
7. Connected Dot Plot: Connected dot plots link data points with lines to illustrate trends over time or sequences. This form is often utilized to represent temporal data, such as the progress of a variable over a time period.
8. Dumbbell Dot Plot: Dumbbell dot plots compare two time points or conditions by connecting paired data points with lines. They clearly visually compare changes or differences between the two time points or conditions.
Example for dot plot:
Here are the students’ test scores: 75, 82, 90, 68, 78, 88, 92, 70, 85, 76, 82, 89, 95, 78, 72, 84, 88, 80, 85, 93.
Step-by-Step Analysis:
Create the Dot Plot: On a number line, plot each test score using a dot above the corresponding value. If multiple students scored the same, stack the dots vertically.
Observe Dot Density: Notice the density of dots at different score values. The more densely packed the dots, the higher the frequency of scores around that value.
Identify Central Tendency: Examine where the dots are most concentrated. In this case, scores between 80 and 85 have the highest density, suggesting a central tendency in that range.
Analyze Spread and Variability: Notice the spread of the dots along the axis. Scores are spread from around 68 to 95. The range of scores indicates variability in performance.
Identify Outliers: Look for dots that stand out from the main cluster. You might notice an outlier at 95, indicating an exceptional score.
Contextual Interpretation: Considering this is a test score analysis, you can conclude that most students scored between 80 and 85, with an outlier at 95. This information might prompt you to investigate what contributed to the outlier’s high score and whether any interventions are needed for students scoring below the average.
Comparative Analysis (if applicable): If you have other classes’ test scores, you could create dot plots for each class and compare their distributions, central tendencies, and outliers to identify any trends or differences.
Draw Conclusions and Communicate: Based on your analysis, you can conclude that the majority of students scored around 80-85. However, there is one outstanding score of 95. This information informs your understanding of student performance and guides any necessary actions.
Do You Stack Up Against the Best?
If you have 30 minutes, try our free diagnostics test and assess your skills.
Dot Plots FAQS
What is a dot plot?
A dot plot is a simple data visualization method that uses dots to represent individual data points along a numerical axis. The density of dots at specific values provides insights into the distribution, frequency, and patterns within a dataset.
How do I create a dot plot?
To create a dot plot, you need a numerical axis and the dataset you want to visualize. Place a dot above the corresponding value on the axis for each data point. If multiple data points share the same value, stack the dots vertically.
What insights can I gain from a dot plot?
Dot plots provide insights into:
- Frequency of data values.
- The central tendency of the data distribution.
- Spread and variability of the data.
- Presence of outliers or exceptional data points.
How can I identify outliers in a dot plot?
Outliers appear as individual dots that stand apart from the main cluster. These are data points that significantly differ from the majority of the data.
What is the difference between a dot plot and a scatter plot?
A dot plot is used for a single variable and represents the frequency and distribution of values along a single axis. On the other hand, a scatter plot is used for two variables. It shows the relationship between them with individual data points as dots.
What are the advantages of using a dot plot?
Dot plots offer simplicity, clarity, and effectiveness in representing data distributions. They are particularly useful for small to moderate datasets, providing an easy-to-understand visualization of data patterns.

Gloria Mathew writes on math topics for K-12. A trained writer and communicator, she makes math accessible and understandable to students at all levels. Her ability to explain complex math concepts with easy to understand examples helps students master math. LinkedIn
Affordable Tutoring Now Starts at Just $22.49
eTutorWorld offers affordable one-on-one live tutoring over the web for Grades K-12. We are also a leading provider of Test Prep help for Standardized Tests (SCAT, CogAT, MAP, SSAT, SAT, ACT, ISEE, and AP).
What makes eTutorWorld stand apart are: flexibility in lesson scheduling, quality of hand-picked tutors, assignment of tutors based on academic counseling and diagnostic tests of each student, and our 100% money-back guarantee.
Whether you have never tried personalized online tutoring before or are looking for better tutors and flexibility at an affordable price point, schedule a FREE TRIAL Session with us today.
*There is no purchase obligation or credit card requirement
Grade 6 Science Worksheets
- Inquiry process
- Nature of Science
- Scientific Inquiry
- Inquiry, Analysis and Problem Solving
- Ethical Practices
- Science and Society
- Biotic and Abiotic Factors
- Impact of Organisms
- Adaptation
- Spheres of Earth
- Natural Resources
- Environmental Issues
- Conservation of Earth
- Understanding Technology
- Abilities To Do Technological Design
- Structure of Earth
- Solar System
- Rocks and Fossils
- Earth Systems
- Plate Tectonics
- Evolution
- Magnetic Field of Earth
- Geologic Time
- Materials and Processes That Shape a Planet
- Astronomy
- Ecology
- Energy
- Kinetic and Potential Energy
- Energy Transfer
- Matter and its Structure
- States of Matter
- Physical and Chemical Changes
- Force and Motion
- Electricity and Magnetism
- Wave Interactions
- Sound
- Light
- Introduction to Life Science
- The Origin & History of Life On Earth
- Plant and Animal Cells
- Parts of a Cell
- The Cell Cycle
- How Living Organisms Get Energy
- Classification of Organisms
- How Plants Grow & Reproduce
- The Human Respiratory System
- The Human Cardiovascular System
- The Human Digestive System
- The Human Endocrine Systems
- The Human Nervous System
- The Human Muscular System
- The Human Skeletal System
IN THE NEWS

Our mission is to provide high quality online tutoring services, using state of the art Internet technology, to school students worldwide.
Online test prep and practice
SCAT
SSAT
ISEE
PSAT
SAT
ACT
AP Exam
Science Tutoring
Physics Tutoring
Chemistry Tutoring
Biology Tutoring
Math Tutoring
Pre-Algebra Tutoring
Algebra Tutoring
Pre Calculus Tutoring
Calculus Tutoring
Geometry Tutoring
Trigonometry Tutoring
Statistics Tutoring
Quick links
Free Worksheets
Fact sheet
Sales Partner Opportunities
Parents
Passive Fundraising
Virtual Fundraising
Our Expert Tutors
Safe and Secure Tutoring
Interactive Online Tutoring
After School Tutoring
Elementary School Tutoring
Middle School Tutoring
High School Tutoring
Home Work Help
Math Tutors New York City
Press
©2022 eTutorWorld Terms of use Privacy Policy Site by Little Red Bird
©2022 eTutorWorld
Terms of use
Privacy Policy
Site by Little Red Bird