Indefinite Integrals

In Physics, we know that the rate of change of displacement is velocity.

Now, if we are given the velocity of an object at any time i.e. any given instant, can we determine the displacement of that object at that instant.

Similarly, the rate of change of velocity is acceleration. If acceleration at any given time is known to us, can we identify its velocity?

Differentiation helps us to find the rate of change of any quantity with respect to another. Similarly, we need to reverse the process to find the answers to the above questions.

The answers to these questions can be given by what is called Integration.

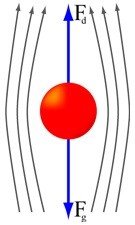
Figure 1: https://commons.wikimedia.org/wiki/File:Terminal_Velocity.png

Here, we introduce the concept of Integration.

Integration is the inverse process of differentiation.
In Differentiation, we are given a function f(x) & we find its derivative i.e. f ‘ (x).
In Integration, we are given the derivative f ‘ (x) of a function f(x). We have to find the original function i.e. f(x) or the anti derivative.

This process is called anti or Integration.

Indefinite Integral

Let F(x) be function which is differentiable in an interval, I. f(x) is a function such

that $\frac{d}{dx}$ (F(x))= F ‘(x)=f(x), x $\in$ I . Then we have,

$\int {}$ f(x) dx = F(x) where, $\int {}$ f(x)dx denotes the family or class of anti derivatives which is read as Indefinite integral of f with respect to x.

Note: C is a constant called the Constant of Integration.

For every distinct value of C, we get a different member of the family. Hence, it is called Indefinite (which is not fixed/certain) integral.

Properties of Indefinite Integrals

$\int {}$ f ‘ (x)dx= f(x)+C
$\int {}$ [f(x)+g(x)]dx = $\int {}$ f(x)dx + $\int {}$ g(x)dx
$\int {}$ [f(x)-g(x)]dx = $\int {}$ f(x)dx – $\int {}$ g(x)dx
$\int {}$ kf(x)dx = k $\int {}$ f(x)dx + C, where k is any non zero real number.
. $\int {}$ [ $k_{{1}}$ f(x) + $k_{{2}}$ g(x)]dx = $k_{{1}}$ $\int {}$ f(x)dx + $k_{{2}}$ $\int {}$ g(x)dx .

Examples

Now let’s consider some examples on indefinite integrals.

Example 1: Find the anti-derivative i.e. Integral of f(x) = $x^8$ + 3x with respect to x.

$\int {}$ ( $x^8$ + 3x)dx = $\int {}$ ( $x^8$ )dx + 3 $\int {}$ ( $x^1$ )dx

= $\frac{ x^{{8+1}}}{8+1}$ +3 $\left ( \frac{ x^{{1+1}}}{1+1} \right )$ +C = $\frac{x^9}{{9}}$ + 3 $\left ( \frac{x^2}{{2}} \right )$ +C

where C is the constant of Integration.

Example 2: Evaluate: $\int {}$ (7Sin x – 3Cos x)dx

$\int {}$ (7Sin x-3Cos x)dx = 7 $\int {}$ Sin xdx – 3 $\int {}$ Cos xdx

= 7(-Cos x) – 3(Sin x) +C = -7Cos x – 3Sin x+C

Example 3: Evaluate: $\int {}$ $x^2$ $\left (1- \frac{1}{x^3} \right )$ dx .

$\int {}$ $x^2$ $\left (1- \frac{1}{x^3} \right )$ dx = $\int {}$ $\left (x^2- \frac{1}{x} \right )$ dx

= $\int {}$ $x^2$ dx – $\int {}$ $\frac{1}{x}$ dx = $\frac{ x^{{2+1}}}{2+1}$ – log|x|+ C = $\frac{x^3}{3}$ – log|x|+C

Example 4: Evaluate: $\int {}$ $\frac{3+4Cos x}{Sin^2x}$ dx

$\int {}$ $\frac{3+4Cos x}{Sin^2x}$ dx = $\int {}$ $\left ( \frac{3}{Sin^2x}+ 4\frac{Cos x}{Sin^2 x} \right )$ dx

= $\int {}$ (3 $Cosec^2$ x + 4CosecxCotx)dx =3 $\int {}$ $Cosec^2$ xdx+4 $\int {}$ CosecxCotxdx .

= -3Cotx -4Cosecx + C

Example 5: Evaluate: $\int {}$ (3 $x^2$ -2Sin x+7 $\sqrt{x}$ )dx

$\int {}$ (3 $x^2$ -2Sin x+7 $\sqrt{x}$ )dx

=3 $\int {}$ $x^2$ dx -2 $\int {}$ Sin xdx+7 $\int {}$ $x^{1/2}$ dx=3 $\frac{ x^{{2+1}}}{2+1}$ -2(-Cosx) +7 $\frac{ x^{{\frac{1}{2}+1}}}{\frac{1}{2}+1}$ +C

= $x^{3}$ +2Cosx + $\frac{14}{3}$ $x^{\frac{3}{2}}$ +C= $x^{3}$ + 2Cosx+ $\frac{14}{3}$ x $\sqrt{x}$ +C

Check Point

Evaluate the following Integrals –

$\int {}$ (15 $x^{14}$ +20)dx
$\int {}$ (7Cosx – 3 $Sec^{2}x$ )dx
$\int {}$ (7 $e^{x}$ + 34 $x^{16}$ +17)dx
$\int {}$ (x-1)(x-2)dx
$\int {}$ $\left ( \sqrt{x}-\frac{1}{\sqrt{x}} \right )$ dx

Answer Key

$\int {}$ (15 $x^{14}$ +20)dx= $x^{15}$ + 20x +C
$\int {}$ (7Cosx – 3 $Sec^{2}x$ )dx = 7Sin x – 3Tan x+C
$\int {}$ (7 $e^{x}$ + 34 $x^{16}$ +17)dx = 7 $e^{x}$ +2 $x^{17}$ +17x+C
$\int {}$ (x-1)(x-2)dx= $\frac{x^3}{3}$ – $\frac{3}{2}$ $x^2$ + 2x + C
$\int {}$ $\left ( \sqrt{x}-\frac{1}{\sqrt{x}} \right )$ dx = $\frac{3}{2}$ $x^{3/2}$ – 2 $x^{1/2}$ +C .

Personalized Online Tutoring

eTutorWorld offers affordable one-on-one live tutoring over the web for Grades 2-12, Test Prep help for Standardized tests like SCAT, CogAT, SSAT, SAT, ACT, ISEE and AP. You may schedule online tutoring lessons at your personal scheduled times, all with a Money-Back Guarantee. The first one-on-one online tutoring lesson is always FREE, no purchase obligation, no credit card required.

For answers/solutions to any question or to learn concepts, take a FREE Demo Session.

Schedule a Free Session

No credit card required, no obligation to purchase.
Just schedule a FREE Sessions to meet a tutor and get help on any topic you want!

Pricing for Online Tutoring

Tutoring Package	Validity	Grade (1-12), College
5 sessions	1 Month	$139
1 session	1 Month	$28
10 sessions	3 months	$269
15 sessions	3 months	$399
20 sessions	4 months	$499
50 sessions	6 months	$1189
100 sessions	12 months	$2249