Rational and Irrational Numbers
Rational and Irrational Numbers
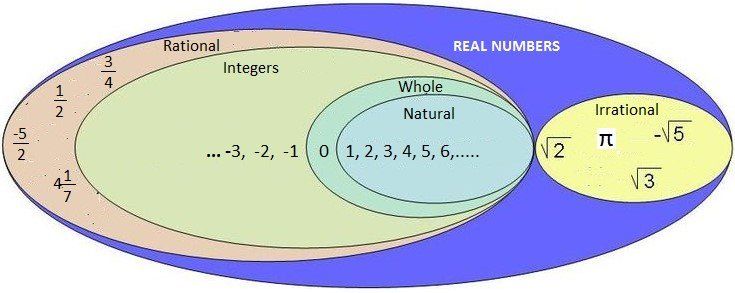
Rational Numbers
Any number that can be expressed in the form where p, q are integers and q
0 is called a rational number. p is called the numerator and q the denominator of the rational number.
Any integer can be expressed as a rational number. For example, 5 can be written as in the rational form.
The numbers ,
,
,
,
,
,… are all rational numbers
All fractions are rational numbers. All integers are also rational numbers. There are many rational numbers which are neither fractions nor integers.
Rational numbers can also be represented on the number line.
Example: Which of the following is (are) rational number(s)?
2, ,
, -9,
It can be seen that apart from, all other numbers can be written in the form
.
So, except, all the given numbers are rational numbers.
Irrational Numbers
All numbers which are not rational are called irrational numbers, which means all the numbers that cannot be put in the form where p, q are integers and q
0 are called ‘irrational numbers’.
Any rational number will be a terminating decimal or a repeating decimal. For example, = 0.875(terminating decimal)
= 0.41666…(repeating decimal)
An irrational number is a decimal that is neither terminating nor repeating.
The square roots of 2, 3, 5, 6, 7, 8 are all irrational numbers.
Example: Which of the following is NOT irrational?
and
are irrational numbers.
= 4, which is a rational number.
=
= 2, which is also a rational number.
So, and
are NOT irrational numbers.
Answer key
- Yes
- π
- 0 is a rational number because we can write it as
,
, which implies that 0 is a rational number.
- (A)
eTutorWorld Understands Math Tutoring | Online Math Worksheets are Important Tools
Understanding graphs, charts, and opinion polls in a newspaper, for calculating house and car payments, and for choosing a long-distance telephone service are impossible without strong math skills …and the only way to develop strong math skills is by constant practice.
‘Practice makes a man perfect’ holds true for no other field better than for math. A middle or high school student must set aside a minimum of an hour for math every day. Other than textbooks, worksheets help you revise and understand concepts better.
Our expert tutors prepare online maths worksheets that are age and grade-appropriate. Grade-wise math worksheets for Elementary Math, Arithmetic, Pre-Algebra, Algebra, Geometry, Trigonometry, Statistics, Pre-Calculus and Calculus can be solved to improve math skills, to get ahead or to even catch up.
You may download these FREE online math worksheets in the PDF format, and then print and email us their solutions for a free evaluation and analysis by eTutorworld’smath expert tutors.
You may solve these worksheets by yourself or with your peers while studying together.
The Answer Key at the end of each worksheet allows for a self-evaluation.
Personalized Online Tutoring
eTutorWorld offers affordable one-on-one live tutoring over the web for Grades K-12, Test Prep help for Standardized tests like SCAT, CogAT, MAP, SSAT, SAT, ACT, ISEE and AP. You may schedule online tutoring lessons at your personal scheduled times, all with a Money-Back Guarantee. The first one-on-one online tutoring lesson is always FREE, no purchase obligation, no credit card required.
For answers/solutions to any question or to learn concepts, take a FREE CLASS.
No credit card required, no obligation to purchase.
Just book a free class to meet a tutor and get help on any topic you want!
Pricing for Online Tutoring
| Tutoring Package | Validity | Grade (1-12), College |
|---|---|---|
| 5 sessions | 1 Month | $139 |
| 1 session | 1 Month | $28 |
| 10 sessions | 3 months | $269 |
| 15 sessions | 3 months | $399 |
| 20 sessions | 4 months | $499 |
| 50 sessions | 6 months | $1189 |
| 100 sessions | 12 months | $2249 |
Arithemetic & Pre Algebra
The Number System
1) Rational and Irrational Numbers
2) Compare Irrational Numbers
3) Fractions and Repeating Decimals
4) Approximating Square Roots
Algebra
Expressions and Equations
1) Exponents and their properties
2) Square roots and Cube roots
3) Scientific Notation – addition, subtraction, division and multiplication
4) Equations with variables on both sides
5) Linear equations with one, zero, or infinite solutions
6) Age Word Problems
7) Solve systems of linear equations with elimination and substitution
8) Word Problems on System of Linear Equations
Geometry
1) Parallel Lines
2) Congruent Angles
3) Finding Angle Measures
4) Pythagorean Theorem
5) Distance Formula
6) Word problems on Volume of Cones, Cylinders, and Spheres
Statistics and Probability
IN THE NEWS

Our mission is to provide high quality online tutoring services, using state of the art Internet technology, to school students worldwide.
Online test prep and practice
SCAT
CogAT
SSAT
ISEE
PSAT
SAT
ACT
AP Exam
Science Tutoring
Physics Tutoring
Chemistry Tutoring
Biology Tutoring
Math Tutoring
Pre-Algebra Tutoring
Algebra Tutoring
Pre Calculus Tutoring
Calculus Tutoring
Geometry Tutoring
Trigonometry Tutoring
Statistics Tutoring
Quick links
Free Worksheets
Fact sheet
Sales Partner Opportunities
Parents
Passive Fundraising
Virtual Fundraising
Our Expert Tutors
Safe and Secure Tutoring
Interactive Online Tutoring
After School Tutoring
Elementary School Tutoring
Middle School Tutoring
High School Tutoring
Home Work Help
Math Tutors New York City
Press
©2022 eTutorWorld Terms of use Privacy Policy Site by Little Red Bird
©2022 eTutorWorld
Terms of use
Privacy Policy
Site by Little Red Bird







